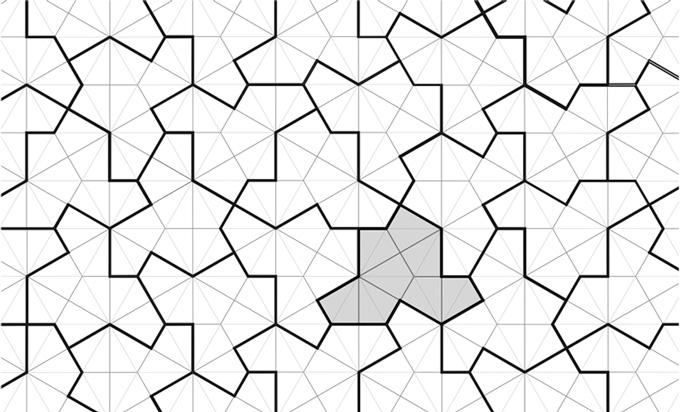
A 13-sided shape known as “the hat” has mathematicians tipping their caps.
It’s the first true example of an “einstein,” a single shape that forms a special tiling of a plane: Like bathroom floor tile, it can cover an entire surface with no gaps or overlaps but only with a pattern that never repeats.
“Everybody is astonished and is delighted, both,” says mathematician Marjorie Senechal of Smith College in Northampton, Mass., who was not involved with the discovery. Mathematicians had been searching for such a shape for half a century. “It wasn’t even clear that such a thing could exist,” Senechal says.
Although the name “einstein” conjures up the iconic physicist, it comes from the German ein Stein, meaning “one stone,” referring to the single tile. The einstein sits in a weird purgatory between order and disorder. Though the tiles fit neatly together and can cover an infinite plane, they are aperiodic, meaning they can’t form a pattern that repeats.
With a periodic pattern, it’s possible to shift the tiles over and have them match up perfectly with their previous arrangement. An infinite checkerboard, for example, looks just the same if you slide the rows over by two. While it’s possible to arrange other single tiles in patterns that are not periodic, the hat is special because there’s no way it can create a periodic pattern.
Identified by David Smith, a nonprofessional mathematician who describes himself as an “imaginative tinkerer of shapes,” and reported in a paper posted online March 20 at arXiv.org, the hat is a polykite — a bunch of smaller kite shapes stuck together. That’s a type of shape that hadn’t been studied closely in the search for einsteins, says Chaim Goodman-Strauss of the National Museum of Mathematics in New York City, one of a group of trained mathematicians and computer scientists Smith teamed up with to study the hat.
It’s a surprisingly simple polygon. Before this work, if you’d asked what an einstein would look like, Goodman-Strauss says, “I would’ve drawn some crazy, squiggly, nasty thing.”
Mathematicians previously knew of nonrepeating tilings that involved multiple tiles of different shapes. In the 1970s, mathematician Roger Penrose discovered that just two different shapes formed a tiling that isn’t periodic (SN: 3/1/07). From there, “It was natural to wonder, could there be a single tile that does this?” says mathematician Casey Mann of the University of Washington Bothell, who was not involved with the research. That one has finally been found, “it’s huge.”

Other shapes have come close. Taylor-Socolar tiles are aperiodic, but they are a jumble of multiple disconnected pieces — not what most people think of as a single tile. “This is the first solution without asterisks,” says mathematician Michaël Rao of CNRS and École Normale Supérieure de Lyon in France.
Smith and colleagues proved that the tile was an einstein in two ways. One came from noticing that the hats arrange themselves into larger clusters, called metatiles. Those metatiles then arrange into even larger supertiles, and so on indefinitely, in a type of hierarchical structure that is common for tilings that aren’t periodic. This approach revealed that the hat tiling could fill an entire infinite plane, and that its pattern would not repeat.
The second proof relied on the fact that the hat is part of a continuum of shapes: By gradually changing the relative lengths of the sides of the hat, the mathematicians were able to form a family of tiles that can take on the same nonrepeating pattern. By considering the relative sizes and shapes of the tiles at the extremes of that family — one shaped like a chevron and the other reminiscent of a comet — the team was able to show that the hat couldn’t be arranged in a periodic pattern.
While the paper has yet to be peer-reviewed, the experts interviewed for this article agree that the result seems likely to hold up to detailed scrutiny.
Nonrepeating patterns can have real-world connections. Materials scientist Dan Shechtman won the 2011 Nobel Prize in chemistry for his discovery of quasicrystals, materials with atoms arranged in an orderly structure that never repeats, often described as analogs to Penrose’s tilings (SN: 10/5/11). The new aperiodic tile could spark further investigations in materials science, Senechal says.
Similar tilings have inspired artists, and the hat appears to be no exception. Already the tiling has been rendered artistically as smiling turtles and a jumble of shirts and hats. Presumably it’s only a matter of time before someone puts hat tiles on a hat.
And the hat isn’t the end. Researchers should continue the hunt for additional einsteins, says computer scientist Craig Kaplan of the University of Waterloo in Canada, a coauthor of the study. “Now that we’ve unlocked the door, hopefully other new shapes will come along.”