Estimating resilience in complex systems
Resilience is an important concept in the study of critical transitions and tipping points in complex systems and is defined by the size of the disturbance that a system can endure before tipping into an alternative stable state. Nevertheless, resilience has proved resistant to measurement. Arani et al. show how the mathematical concept of mean exit time, the time it takes for a system to cross a threshold, can help to solve this problem and characterize the resilience of complex systems. They derived a model approach to estimate exit time from time series data and applied it to examples from a grazed plant population model, lake cyanobacterial data, and Pleistocene-Holocene climate data. This approach may improve our understanding of the dynamical properties of complex systems under threat.
Science, aay4895, this issue p. eaay4895
Structured Abstract
INTRODUCTION
Financial markets may collapse, rainforest can shift to savanna, a person can become trapped in a depression, and the Gulf Stream can come to a standstill. Such critical transitions may happen in complex systems when resilience is low, allowing a perturbation to trigger self-propelled change toward the contrasting state. An intuitive way of visualizing this is to depict the system as a marble in a cup. If the cup is shallower, resilience is lower, and it becomes easier to flip the marble out. Resilience may sometimes be inferred from autocorrelation and variance of time series because they carry information about recovery rates from small perturbations, thus reflecting the slope of the attraction basin. However, although differences in such indicators may reflect differences in resilience, they cannot be interpreted in an absolute sense. The alternative—measuring resilience as the maximum perturbation that a system can take—may seem more attractive. However, this classical ecological definition of resilience assumes that the system is affected by distinct, isolated perturbations. In reality, most systems are perturbed instead by a never-ending natural regime of shocks and fluctuations. Thus, they rarely recover from a perturbation before the next one comes. As a result, the imaginary marble in the cup keeps wandering around, and occasionally a sequence of small but synergistic “perturbations” will push the system across the border of the basin of attraction. How then can we characterize the resilience of such systems in a useful and practical way?
RATIONALE
We propose to use “life expectancy” as a measure of resilience, which can be formalized as the mean exit time from an attraction basin (i.e., the expected time required to cross the border of the basin). This approach has the advantage of taking the natural variability of real complex systems explicitly into account. If we have many observations of shifts, we can calculate the mean exit time simply as the average time the system spends in each given state. However, such data are rare. Moreover, the permanent fluctuations also contain information about the system, which would be lost if one considers only the rare occasions at which a shift occurs. In the approach we outline, this information is used as we infer the deterministic and stochastic components of the underlying dynamical system from observed fluctuations. Applying techniques from statistical mechanics, we show how one can subsequently use the reconstructed empirical model to compute the expected mean exit time for each basin of attraction.
RESULTS
After using model-generated data to demonstrate the method, we applied it to two examples spanning very different time scales: the rapid dynamics of Cyanobacteria in a lake, and the much slower alternations between cold glacial and warmer interstadial regimes in the climate (the so-called Dansgaard-Oeschger events). For both time series, we show that one can reconstruct a model that has two alternative attractors. We estimated the mean exit time for each of these attractors, and we show how one can analyze the uncertainty of this estimate.
A major challenge is that the approach requires high-resolution time series that cover dynamics across both basins of attraction. Such information can be obtained from a single long time series if it includes enough shifts between attraction basins. Alternatively, one may piece the required data together using shorter time series from sets of similar systems.
CONCLUSION
Characterizing resilience as the estimated life expectancy—of a rainforest, a coral reef, or the thermohaline circulation—is a natural and intuitively straightforward way to go. The high-resolution time series required for the approach we outline are still relatively rare. However, possibilities for automated sensing are rapidly expanding in fields as diverse as biomedicine, climate science, ecology, and financial markets. Against this background, the technique for estimating mean exit times is an exciting additional tool to anticipate critical transitions in the many complex systems on which humanity depends.
Time series in this simulated example (B) reflect the underlying stability landscape (A) as well as the regime of stochastic perturbations. Given sufficiently long time series, we can estimate how the average change depends on the state (C), revealing attracting (black dots) and repelling (open dot) equilibria. Meanwhile, variance in the observed change allows estimation of the role of stochasticity (D). The resulting empirical model [based on (C) and (D)] can be used to compute the probability distribution of states (E), the mean exit time given any initial state (F), and the weighted mean exit time for the alternative basins of attraction [horizontal lines in (F)]. The shaded areas in (C), (D), and (F) are the estimated 95% confidence intervals.
” data-icon-position=”” data-hide-link-title=”0″>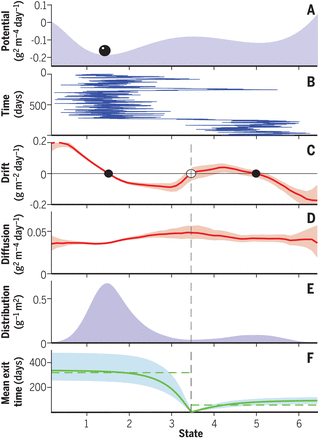

Time series in this simulated example (B) reflect the underlying stability landscape (A) as well as the regime of stochastic perturbations. Given sufficiently long time series, we can estimate how the average change depends on the state (C), revealing attracting (black dots) and repelling (open dot) equilibria. Meanwhile, variance in the observed change allows estimation of the role of stochasticity (D). The resulting empirical model [based on (C) and (D)] can be used to compute the probability distribution of states (E), the mean exit time given any initial state (F), and the weighted mean exit time for the alternative basins of attraction [horizontal lines in (F)]. The shaded areas in (C), (D), and (F) are the estimated 95% confidence intervals.
Abstract
Ecological resilience is the magnitude of the largest perturbation from which a system can still recover to its original state. However, a transition into another state may often be invoked by a series of minor synergistic perturbations rather than a single big one. We show how resilience can be estimated in terms of average life expectancy, accounting for this natural regime of variability. We use time series to fit a model that captures the stochastic as well as the deterministic components. The model is then used to estimate the mean exit time from the basin of attraction. This approach offers a fresh angle to anticipating the chance of a critical transition at a time when high-resolution time series are becoming increasingly available.


