There was a secret inside the envelope in the hands of Stephan Schlamminger, one of the world’s leading experts in experimental tests of gravity. He appeared to be on the verge of opening the envelope during a presentation at the April 2022 meeting of the American Physical Society, to read a number that would reveal whether his latest efforts in a lifelong passion had been a success.
Schlamminger, of the National Institute of Standards and Technology in Gaithersburg, Md., sought to measure Newton’s gravitational constant. The secret number in the envelope was a kind of code — an intentional and specific error inserted into his NIST experiment to obscure the measurement as it progressed. Only one person knew the number. And that person was not Schlamminger.
Without access to it, he couldn’t know what the experiment had found. Schlamminger had imposed the secrecy on himself to protect against bias in the experiment, including the unconscious bias that can beset even the best experimentalists. It was extra precaution to ensure the integrity of an experiment that could help untangle mysterious discrepancies in measurements of the constant, known as G, that have crept up over the last few decades.
G, often called “big G” (to distinguish it from “g,” which depends on G and is the special case of the acceleration of gravity near the surface of the Earth), reflects the strength of gravity between any things with mass. It determines the orbits of planets and galaxies, and describes the force that pulls you to the ground. Nobody knows how to predict from theory what the actual value of G should be, says Clive Speake, a physicist at the University of Birmingham in England who developed the instrument that Schlamminger is using at NIST.
It’s also very difficult to measure. After two centuries of improved precision, recent measurements of G are troubling. A handful of labs around the world have turned up values that disagree (SN Online: 4/30/15). The scattered values could be a sign of trouble with the measurement techniques among various groups, or there might be a more intriguing aspect.
“There’s this haunting elephant in the room which suggests that maybe there’s something going on that we don’t understand,” Speake says. “If the measurements are right, then it could be the greatest discovery since Newton.”
Like so many science presentations in the time of COVID-19, Schlamminger’s reveal was set to be virtual. Presumably other physicists and science reporters around the world were, like me, hunched over screens waiting to see what the secret number would tell us about G.
The time had come to tear open the envelope. But the video feed stopped. The big reveal had been canceled. Puzzling discrepancies in the measurements meant the numbers could not be trusted. The envelope would remain sealed for at least another year as Schlamminger went back to the lab to take another shot at one of the most challenging measurements in physics.
What is Newton’s gravitational constant?
Newton’s gravitational constant is a misnomer. Though Isaac Newton developed his theory of gravity in the 17th century, he didn’t think in terms of G. He was primarily interested in how the force moved objects. Apples falling, planets in orbit and the surprisingly squashed shape of the Earth are just a few of the countless phenomena that Newton’s theory explained, all without explicitly mentioning G. The constant, named for Newton two centuries later, was instead wrapped into the masses involved.
We now know that Newton’s theory is only an approximation of Einstein’s more encompassing version of gravity, the general theory of relativity. It took Einstein’s theory to explain the intense gravity of black holes and the warping of space and time. Still, back here on Earth, it’s Newton’s theory of gravity that concerns Schlamminger and others wanting to measure G.
The force of gravity depends on three factors: the masses involved, the distances between the masses and G. While the masses and distances differ depending on whether you’re considering the forces between you and the Earth, for example, or a planet orbiting the sun, G is always the same. Along with the masses of elementary particles, the charge on an electron and the speed of light, G is one of dozens of constants crucial to science today (SN: 11/12/16, p. 24).
G, though, stands out from the rest. It’s one of the oldest recorded constants — only the speed of light was measured earlier. Yet despite hundreds of elegant experiments since British physicist Henry Cavendish first measured it 225 years ago, G remains among the least precisely known of the fundamental constants.
And in a way, our understanding of G has only worsened in recent decades as new, incompatible measurements have come in.
Recent measurements of big G don’t agree
When Cavendish performed his first measurements of the force of gravity in a lab, he relied on a set of lead spheres. Two of them were hanging at either end of a rod 6 feet (about 2 meters) long, and the whole contraption was dangled from a wire. He then placed larger lead balls nearby and measured the forces between the spheres by tracking how the hanging rod twisted on the wire. Although Cavendish was primarily interested in figuring out the Earth’s density, a little manipulation of his results shows that he effectively measured G for the first time. He got a value about 1 percent higher than today’s generally accepted value.
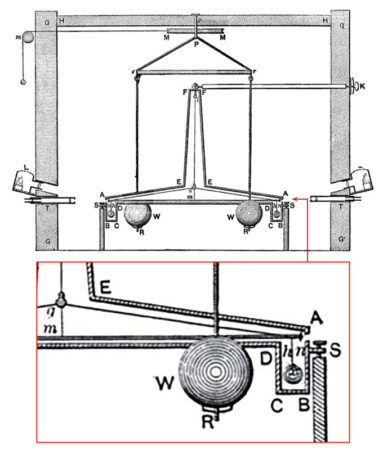
Many of the modern-day G experiments are refined versions of Cavendish’s setup. That includes the one Schlamminger uses. Instead of lead spheres, Schlamminger’s system has precisely machined copper cylinders. Four 1.2-kilogram cylinders, known as test masses, rest on a disk that hangs from a metallic ribbon. The gravitational attraction between the suspended cylinders and four larger, roughly 11-kilogram copper cylinders nearby causes the disk to rotate on the ribbon. Schlamminger calls the heavy cylinders source masses. He’s also done the experiment with a set of source masses made of sapphire crystal to see if G depends on the materials involved (it shouldn’t). Where Cavendish used a large wooden box to protect his apparatus from stray breezes, Schlamminger relies on a vacuum chamber to eliminate the air almost entirely.
Conceptually, the experiment running at NIST is the same as the one Cavendish used. But modern experiments offer much higher precision.
Cavendish’s experiments returned a value of 6.74 x 10-11 cubic meters per kilogram-second squared. The number is correct to about one part in 100. These days, the accepted value is 6.67430 x 10-11 with an uncertainty of about a part in 50,000, which means an error of plus or minus 0.00002 x 10-11. Some experiments have reached similar precision by relying on pendulums swinging near heavy masses instead of twisting wires.
But as precision increased, a new problem came along. Measurements over the last 20 years from various groups don’t agree. It’s as if G is slightly different in different places and at different times in a way that experimental error can’t account for. Schlamminger’s apparatus is on loan to NIST from the International Bureau of Weights and Measures, or BIPM, in Sèvres, France, where researchers came up with a value of 6.67554 x 10-11, a clear deviance from the accepted value (SN Online: 9/12/13).
The original G test
Many modern tests of G are based on Henry Cavendish’s original torsion balance design (shown), developed more than two centuries ago. Cavendish hung two small lead spheres at either end of a long rod that was also hanging from a wire. He placed larger lead spheres nearby (inset). How the hanging rod twisted on its wire revealed the force of attraction between the smaller and larger lead spheres. Instead of lead spheres, an ongoing experiment at NIST uses copper cylinders.
The most likely reason for the discrepancies is that there’s something about each experimental system that’s unique. Researchers are keen to track down these sorts of systematic errors. But measuring gravity is tough, both because it’s the weakest of the fundamental forces (gravity is so weak that some modern experiments use tons of material to home in on G) and because everything with mass has gravity. There’s no way to shield the experiments from other sources of gravity, so researchers must attempt to account for the outside influences.
Alternatively, the discrepancies in G could have something to do with where the experiments are taking place. Perhaps the value of G in Sèvres really is 0.04 percent higher than the value of G recently measured in Boulder, Colo., for example. None of the experts contacted for this story think that’s a likely scenario. But in borrowing the BIPM gravity experiment and moving it to NIST’s campus in Maryland, Schlamminger’s efforts should help confirm that G doesn’t vary from place to place. That’s assuming he can work out whatever threw a wrench in his April 2022 reveal.
Why do we care about the precision of big G?
Why do scientists need to measure G to perpetually higher precision anyway?
According to some experts, they don’t. “From a practical point of view, there isn’t a huge benefit to be derived from knowing G better,” says physicist Clifford Will of the University of Florida in Gainesville. Other constants, like the charge on an electron and the speed of light, “play a huge role in all kinds of important technology, whereas G doesn’t, because gravity is so darn weak,” Will says. “By the time gravity matters, on scales ranging from planets to the universe, what matters is G times mass.”
Physicist Claudia de Rham of Imperial College London has a different view. “G governs the strength of the gravitational force. In Newtonian gravity, it tells us how two massive bodies are gravitationally attracted to one another, but in Einstein’s theory of general relativity, this constant communicates how anything in our universe curves the fabric of spacetime.” Getting a better handle on G, she says, might help explain why gravity is so much weaker than electromagnetic forces or the strong nuclear force, which holds the parts of atoms together.
Although general relativity has proved to be one of the most successful and revolutionary theories in history, de Rham points out that its description of gravity may not be complete (SN: 2/13/21, p. 16). “Testing G to higher precision allows us to understand how constant and universal G really is and if there couldn’t be something else beyond Einstein’s theory of general relativity,” de Rham says.
Some researchers speculate that precise measures of G might one day help uncover the solution to one of the deepest mysteries in science: Why doesn’t gravity fit in with quantum physics? The standard model of particle physics is a quantum theory that describes just about everything in the universe, except gravity. Understanding G better, de Rham says, might lead to a quantum version of gravity, which is necessary to blend gravity into the standard model. Such a “theory of everything” has been a dream of physicists since at least Einstein.
For Schlamminger, the motivation is multifaceted. “It’s mostly pure curiosity. And right now, there’s salt in the wounds that the agreement [between experimental groups] is so bad.” The thrill of pushing an extraordinarily difficult experiment just a bit further drives him too. “Why do people climb Mount Everest?” Schlamminger says. “Because it’s there.”
Other approaches to measuring big G
One perennial challenge with Cavendish-style experiments is the wires. To interpret what’s going on with G, researchers have to know how the suspending wires respond to twisting or swaying, and how they change as they age.
Some researchers choose to do away with the pesky wires altogether, instead dropping or tossing things to see how they respond to the pull of nearby masses. The most precise versions of those experiments so far toss supercooled clouds of atoms up in a tower and then allow them to fall back down again. By doing the tossing with various configurations of heavy objects nearby, researchers can see how the gravitational force exerted by those objects affects the atoms’ trajectories. So far, the experiments have come up short of the most precise suspended-mass experiments by a factor of around 10, achieving a precision of one part in 5,000.
A recent experiment designed with other purposes in mind also dispensed with wires. The Laser Interferometer Space Antenna, or LISA, Pathfinder mission was a proof-of-principle test for a different type of gravity experiment. It was designed to show that it’s possible to precisely measure the distance between objects in space — key to building a space-based gravitational wave detector (SN Online 12/3/15).
LISA Pathfinder managed to measure the distance between objects well enough to find G to within about one part in 15. That’s crude compared with Cavendish’s precision of one in 100, and much worse than other modern measurements. But it shows that an experiment in space, free of the complication of wires and nearby massive objects like the Earth, has potential to measure G in an entirely new way.
Another shortcoming of Cavendish-like experiments is that they measure forces between objects that are either moving slowly or standing completely still. These experiments can’t say much about whether G stays constant when things are moving quickly.
In experiments deep inside a mountain in Switzerland, mechanical engineering researcher Jürg Dual of ETH Zurich is replacing static masses with vibrating beams or rods rotating like helicopter blades (SN Online: 7/11/22). The motions result in changes in distance between the moving parts and a beam that acts as a detector, which in turn changes the gravitational forces the detector beam feels. The detector beam vibrates like a tuning fork, and the size of those vibrations offers a measure of G.
Unlike conventional experiments, this one could detect whether G depends on motion, which “might be something quite spectacular actually,” Dual says. As for how likely that is, “I’m completely open,” he says.
For now, though, Schlamminger and others using hanging masses much as Cavendish did 225 years ago are still providing the most precise measurements.
There’s no clear answer yet
Schlamminger’s lab on the NIST campus is well below his office. “It’s about four stories underground,” he says. “There is less vibration, it’s easier to stabilize the temperature and the lab floor does not tilt as much. Usually buildings tilt with varying wind load. That is not a problem underground.”
On my visit to NIST a month after the canceled reveal, we head down several flights of stairs and take a walk through a vacant hall before entering a room that has a sticky mat just inside. It’s there to clean dust from your shoes as you go in. Even so, Schlamminger switches to a dedicated pair of shoes he stashes in the lab and gives me covers to slip over the soles of my shoes. Then we pass through another, airtight door to see the gravity experiment on loan to NIST. Things must be tidy when you’re trying to do something as difficult as measuring G.
Dust interfering with the tips of measurement probes could throw off readings of the positions of the cylinders. “A second concern, albeit smaller,” Schlamminger says, “is that dust settling on the source masses will change their mass.”
The G experiment is smaller than Cavendish’s pioneering design. You could fit it on a modest dining table. Here, it sits on a massive slab that minimizes the vibrations that manage to make it down to the lab. The vacuum chamber hides some of the moving parts of the apparatus from view.
Schlamminger is between runs at the moment, but four copper source masses, each about the diameter of a 2-liter soda bottle, are at the ready for the next G measurement. The source masses ride on a carousel outside the vacuum chamber, while the test masses sit on the disk suspended inside the chamber.
In the experimental mode that most closely mimics Cavendish’s experiment, tracking the rotation of the disk as it twists on the suspending ribbon offers a measure of the force between the source and test masses, revealing G. In another mode, Schlamminger determines G by finding the force it takes to prevent the disk from rotating.
A set of sapphire crystal source masses that are the same size as the copper ones are in a case nearby. They can take the place of the copper ones on the carousel to confirm that G is a true constant that doesn’t depend on the materials involved. At roughly twice the mass of the sapphire cylinders, the copper versions provide a better measure of G. Precisely how much each of the source masses weigh, though, Schlamminger doesn’t know. That’s because of the secret number tucked away in the sealed envelope.
“The big M, which is the masses of my big copper masses,” he says, “I have basically asked this mass group at NIST who has measured them to add a random factor.” Any studies he makes of G will be slightly off due to the random factor added to the true masses. Just how far off the measurement is won’t be clear until he opens the envelope. So why didn’t he open it in April 2022?
“I measured big G for like three months solid,” Schlamminger says, before popping open the vacuum chamber to check the placement of the cylinders. “Then I did another big G run, and the number was different. And that’s why I did not open the envelope, because I figured there’s something that I don’t understand.”
It turns out he had prerecorded his talk and expected to reveal the answer in real time at the meeting. He changed his mind before the presentation streamed, which is why the audience was left wondering.
There are hints that changes in the quality of the vacuum that come with opening and closing the experimental chamber are related to the measurement shifts. It’s another factor that Schlamminger says researchers will have to keep in mind if they’re to understand the discrepancies in measurements of Newton’s gravitational constant.
These days, Schlamminger is back at it with another experimental run. But one year on, at the 2023 American Physical Society meeting in Minneapolis, he still wasn’t ready to open the envelope. “I’m very, very careful with it because you can’t unopen the envelope.”
